Trigonometry formula list

Explore essential trigonometry formulas, including sine, cosine, and tangent identities, along with angle sum and difference formulas. These key equations are crucial for solving trigonometric problems in mathematics, helping students and professionals simplify complex calculations and understand relationships between angles and sides in triangles. Trigonometry formula list

You see these formulae in the given link

Trigonometric formulae for class 10

Relation between degree and radian

A circle subtends at the centre an angle whose radian measure is 2π and its degree measure is 360°

Then, 2 π Radian = 360°

⇒ $1 \text{Radian} = \dfrac{360}{2\pi}$ degree

Now, $1 \text{Radian} = \dfrac{180}{\pi} = 57^\circ 16'$ approximately

And $1^\circ = \dfrac{\pi}{180}$ Radian = 0.01746 radian approximately

Relation between arc length, Radius and Angle(In Radian)

Since in a circle of radius r, an arc of length l subtends an angle whose measure is θ(In Radian)

Then, l = r × θ or θ = l/r

Trigonometric Functions

(1) Sin x = 1/Cosec x (2) cosec x = 1/Sin x

(3) Cos x = 1/Sec x (4) Sec x = 1/cos x

(5) Tan x = 1/Cot x (6) Cot x = 1/Tan x

(7) Tan x = Sin x/Cos x (8) Cot x = Cos x/Sinx

(9) (i) Sin²x + Cos²x = 1

(ii) Sin²x = 1 – Cos²x

(iii) Cos²x = 1 – Sin²x

(10)(i) Sec²x = 1 + Tan²x

(ii) Sec²x – Tan²x = 1

(iii) Tan²x = Sec²x – 1

(11) (i) Cosec²x = 1 + Cot²x

(ii) Cosec²x – Cot²x = 1

(iii) Cot²x = Cosec²x – 1

(12) sin x = 0 implies x = nπ, where n is any integer

(13) cos x = 0 implies x = (2n + 1)π/2, where n is any integer

Trigometry value table

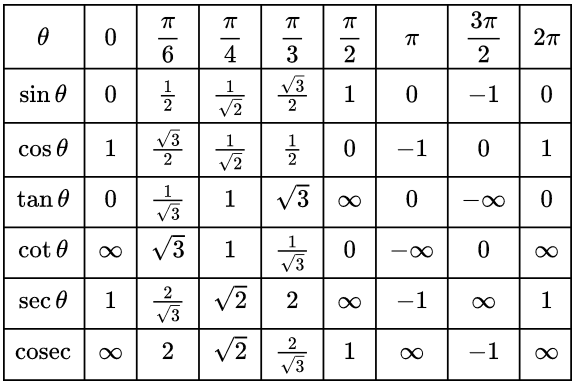
Trigonometry formulae for class 11

Sign of trigonometric functions

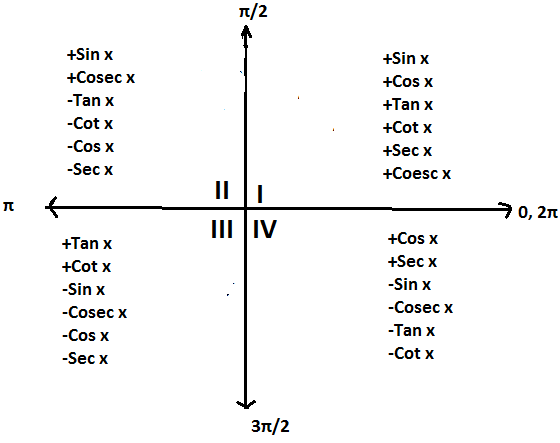
Range of Trigonometric function in quadrant

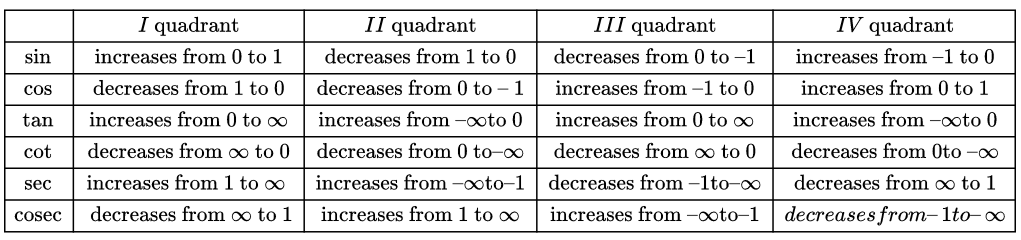
Domain and Range of Trigonometric function

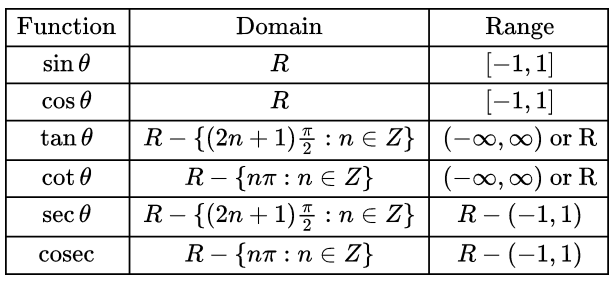
When we add or subtract 2π to any trigonometric function then the value of that function remain unchanged

For example : (i) Sin θ = Sin(2π + θ) (ii) Sin θ = Sin (θ – 2 π)

(iii) Cos 765° =Cos(45 + 2× 360)

⇒ Cos 45 = 1/√2

Trigonometric Functions of Sum and Difference of Two Angles

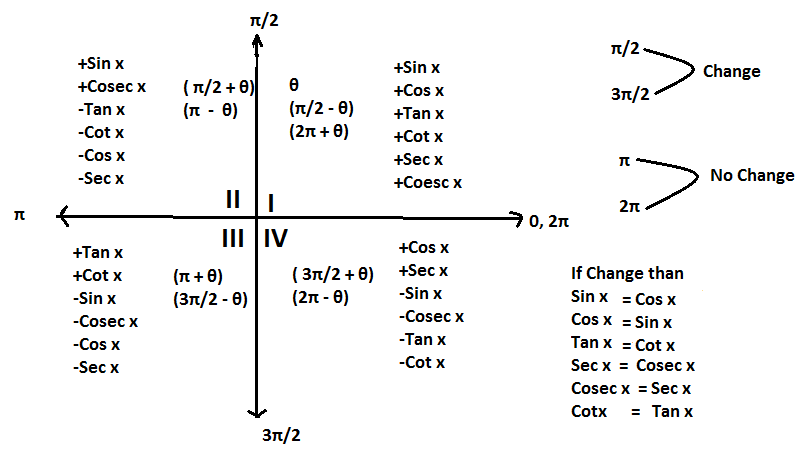
Example: (i) Sin(π/2 – x)

(π/2 – x) lies in Ist quadrant and ‘Sin’ function remain positive in Ist quadrant

Value of Sin x will change to Cos x

Then the value of ‘Sin(π/2 – x) = Cos x’

Some other formulae

1.(i) $\sin(-x) = -\sin x$ (ii) $\cos(-x)= \cos x$

(iii) $\tan(-x) = -\tan x$ (iv) $\cot(-x) = -\cot x$

(v) $\sec(-x) = \sec x$ (vI) $\operatorname{cosec}(-x)= -\operatorname{cosec}x$

2.(i) $\sin(\frac{\pi}{2}-x) = \cos x$ (ii) $\cos(\frac{\pi}{2}-x) = \sin x$

(iii) $\tan(\frac{\pi}{2}-x) = \cot x$ (iv) $\cot(\frac{\pi}{2}-x) = \tan x$

(v) $\sec(\frac{\pi}{2}-x) = \operatorname{cosec} x$ (vi) $\operatorname{cosec}(\frac{\pi}{2}-x) = \sec x$

3. (i) $\sin(\frac{\pi}{2}+x) = \cos x$ (ii) $\cos(\frac{\pi}{2}+x) = -\sin x$

(iii) $\tan(\frac{\pi}{2}+x) = -\cot x$ (iv) $\cot(\frac{\pi}{2}+x) = -\tan x$

(v) $\sec(\frac{\pi}{2}+x) = -\operatorname{cosec} x$ (vi) $\operatorname{cosec}(\frac{\pi}{2}+x) = \sec x$

4.(i) $\sin(\pi - x) = \sin x$ (ii) $\cos(\pi-x) = -\cos x$

(iii) $\tan(\pi - x) = -\tan x$ (iv) $\cot(\pi-x) = -\cot x$

(v) $\sec(\pi - x) = -\sec x$ (vi) $\operatorname{cosec}(\pi-x) = \operatorname{cosec}x$

5. (i) $\sin(\pi +x) = -\sin x$ (ii) $\cos(\pi + x) = -\cos x$

(iii) $\tan (\pi + x) = \tan x$ (iv) $\cot(\pi + x) = \cot x$

(v) $\sec(\pi +x) = -\sec x$ (vi) $\operatorname{cosec}(\pi + x) = -\operatorname{cosec}x$

6. (i) $\sin(\frac{3\pi}{2}-x) = -\cos x$ (ii) $\cos(\frac{3\pi}{2}-x) = -\sin x$

(iii) $\tan(\frac{3\pi}{2}-x) = \cot x$ (iv) $\cot(\frac{3\pi}{2}-x) = \tan x$

(v) $\sec(\frac{3\pi}{2}-x) = -\operatorname{cosec} x$ (vi) $\operatorname{cosec}(\frac{3\pi}{2}-x) = -\sec x$

7.(i) $\sin(\frac{3\pi}{2}+x) = -\cos x$ (ii) $\cos(\frac{3\pi}{2}+x) = \sin x$

(iii) $\tan(\frac{3\pi}{2}+x) = -\cot x$ (iv) $\cot(\frac{3\pi}{2}+x) = -\tan x$

(v) $\sec(\frac{3\pi}{2}+x) = \operatorname{cosec} x$ (vi) $\operatorname{cosec}(\frac{3\pi}{2}+x) = -\sec x$

8.(i) $\sin(2\pi - x) = -\sin x$ (ii) $\cos(2\pi-x) = \cos x$

(iii) $\tan(2\pi - x) = -\tan x$ (iv) $\cot(2\pi-x) = -\cot x$

(v) $\sec(2\pi - x) = \sec x$ (vi) $\operatorname{cosec}(2\pi-x) = -\operatorname{cosec}x$

9.(i) $\sin(2\pi +x) = \sin x$ (ii) $\cos(2\pi + x) = \cos x$

(iii) $\tan (2\pi + x) = \tan x$ (iv) $\cot(2\pi + x) = \cot x$

(v) $\sec(\pi +x) = \sec x$ (vi) $\operatorname{cosec}(2\pi + x) = \operatorname{cosec}x$

10.(i) $\sin(x+y)=\sin x\cos y+\cos x\sin y$

(ii) $\sin(x-y)=\sin x\cos y-\cos x\sin y$

(iii) $\cos(x + y) = \cos x\cos y - \sin x\sin y$

(iv) $\cos (x - y) = \cos x\cos y + \sin x\sin y$

(v) $\tan(x + y) = \dfrac{\tan x + \tan y}{1-\tan x.\tan y}$

(vi) $\tan(x - y) = \dfrac{\tan x - \tan y}{1 + \tan x.\tan y}$

(vii) $\cot(x + y)) = \dfrac{\cot x.\cot y-1}{\cot y + \cot x}$

(viiii) $\cot(x - y) = \dfrac{\cot x.\cot y+1}{\cot y - \cot x}$

11. (i) $\sin 2x =2\sin x\cos x$

(ii) $\sin 2x = \dfrac{2\tan x}{1+\tan^2x}$

12. (i) $\cos 2x = \cos^2x - \sin^2x$

(ii) $\cos 2x = 1 - 2\sin^2x$

(iii) $\cos 2x = 2\cos^2x - 1$

(iv) $\cos 2x = \dfrac{1 - \tan ^2x}{1 + \tan^2x}$

13. $\tan 2x = \dfrac{2\tan x}{1 - \tan^2x}$

14. $\sin 3x = 3\sin x - 4\sin^3x$

15. $\cos 3x = 4\cos^3x - 3\cos x$

16. $\tan 3x = \dfrac{3\tan x - \tan^3x}{1 - 3\tan^2x}$

17. (i) $\sin x =2\sin \frac{x}{2}\cos\frac{x}{2}$

(ii) $\sin x = \dfrac{2\tan \frac{x}{2}}{1+\tan^2\frac{x}{2}}$

18. ( i) $\cos x = \cos^2\frac{x}{2} - \sin^2\frac{x}{2}$

(ii) $\cos x = 1 - 2\sin^2\frac{x}{2}$

(iii) $\cos x = 2\cos^2\frac{x}{2} - 1$

(iv) $\cos x = \dfrac{1 - \tan ^2\frac{x}{2}}{1 + \tan^2\frac{x}{2}}$

19. $\tan x= \dfrac{2\tan \frac{x}{2}}{1 - \tan^2\frac{x}{2}}$

20. (i) $1 + \cos 2x = 2\cos^2x$

(ii) $1-\cos 2x = 2\sin^2 x$

21. (i) $1 +\cos x = 2\cos^2 \frac{x}{2}$

(ii) $1 - \cos x = 2\sin^2\frac{x}{2}$

22. (i) $\sin x + \sin y = 2\sin\dfrac{x + y}{2}\cos \dfrac{x - y}{2}$

(ii) $\sin x - \sin y = 2\cos\dfrac{x + y}{2}\sin \dfrac{x - y}{2}$

(iii) $\cos x + \cos y = 2\cos\dfrac{x + y}{2}\cos \dfrac{x - y}{2}$

(iv) $\cos x - \cos y = -2\sin\dfrac{x + y}{2}\sin \dfrac{x - y}{2}$

23. (i) $2\sin x\cos y = \sin (x + y) +\sin(x - y)$

(ii) $2\cos x \sin y = \sin(x + y) - \sin(x - y)$

(iii) $2\cos x \cos y = \cos(x + y)+\cos(x-y)$

(iv) $2\sin x\sin y = \cos(x -y ) - \cos (x + y)$

Trigonometric formulae for class 10

Class 10 Case study Chapter 8 introduction to Trigonometry