Class 10 Case based problem of Chapter 3 Pair of Linear eq 1

by

Chapter 3:Pair of Linear equation in two variables

Case Based :1

             A book store shopkeeper gives books on rent for reading. He has variety of books in his store related to fiction,  stories and quizzes etc. He takes a fixed charge for the first two days and on addition charge for subsequent day. Amruta paid ₹ 22 for a book and kept for 6 days, while Radhika paid ₹ 16 for keeping the book for 4 days . Assume that the fixed change be ₹ x and additional change(per day) be ₹ y.(Class 10 Case based problem of Chapter 3 Pair of Linear eq 1)

Class 10 Case based problem of Chapter 3 Pair of Linear eq 1
Stack of books on display at the bookstore

Based on the above information, answer any four the following question:

(A) The situation of amount paid by Radhika, is algebraically represented by

(a) x – 4y = 16

(b) x + 4 y = 16

(c) x – 2y = 16

(d) x + 2y = 16

(B) The situation of amount paid by Amruta, is algebraically represented by

(a) x – 2y = 11

(b) x – 2y = 22

(c) x + 4y = 22

(d) x – 4y =11

(C) What are the fixed charges for a book ?

(a) ₹ 9                 (b) ₹ 10

(c) ₹ 13                (d) ₹ 15

(D) What are the additional charges for each subsequent day for a book ?

(a) ₹ 6                   (b) ₹ 5

(c) ₹ 4                    (d) ₹ 3

(E)What is the total amount paid by both, if both of them have kept the book for 2 more days ?

(a) ₹ 35                  (b) ₹ 52

(c) ₹ 50                   (d) ₹ 58

Solution:

(A) Answer (d) x + 2y = 16

Explanation:

Radhika kept book for 4 days and paid ₹ 16.

Hence, x + 2y =16

(B) Answer (c) x + 4y =22

Explanation:

Since Amruta kept book for 6 days and paid ₹ 22

Hence, x + 4y = 22

(C) Answer (b) ₹ 10

Explanation:

From A and B. we have

x + 2y = 16 —–(i)

x + 4y = 22 —–(ii)

Subtracting (i) to (ii) we get

(x + 2y) – (x + 4y) = 16-22

⇒ x + 2y – x-  4y = -6

⇒  -2y = -6

⇒  y = 3

Replacing the value of y in (i)

x + 2y = 16

⇒ x + 2×3 = 16

⇒ x = 16 – 6

⇒ x = 10

Fixed charges = ₹ x = ₹ 10

(D) Answer (d) ₹3

From solution (C) Additional charges for each subsequent day = ₹ y = ₹ 3

(E) Answer (c) ₹ 50

Explanation:

If both of them have kept the book for 2 more days

= 16 +2y + 22 +2 y

= 38 + 4×3

= 38 + 12

=  ₹ 50

Case Based :2

Raman went to a local mela during dussehra. He ate several rural delicacies such as jalebis chaat etc. He also wanted to play the ring game in which a ring is thrown on the items displayed on the table and the balloon shooting game.

Class 10 Case based problem of Chapter 3 Pair of Linear eq 1
Raman went to a local mela during dussehra.
Class 10 Case based problem of Chapter 3 Pair of Linear eq 1
Raman went to a local mela during dussehra.

The cost of three balloon shooting games exceeds the cost of four ring games by ₹ 4. Also, the total cost of three balloon shooting games and four ring games is ₹ 20.

 (A) Taking the cost of one ring game to be ₹ x and that of one balloon game as ₹y, the pair of linear equations describing the above situations are:

(a) -4x – 3y = -4 and 4x + 3y = 20

(b) 4x – 3y = 4 and 4x + 3y = 20

(c) 4x – 3y = -4  and 4x + 3y = 20

(d) -4x + 3y = -4 and 4x + 3y = 20

(B) The cost of one ring game and one balloon game is :

(a) ₹ 2 and ₹ 4

(b) ₹4 and ₹ 2

(c) ₹8 and  ₹2

(d) ₹ 6 and ₹ 3

(C) The points where the line represented by the equation 4x – 3y = -4 intersects the x-axis and y-axis, respectively, are given by:

(a) (1, 0), (0,  4/3)             (b) (-1, 0), (0, 4/3)

(c) (-1, 0), (0, -4/3)            (d) (1, 0), (0, -4/3)

(D) The area of the triangle formed by the two lines and the x- axis is:

(a) 4 sq. units                      (b) 6 sq. units

(c) 8 sq. units                       (d) 12 sq. units

(E) The value of k for which the pair of linear equations – x + y = -1, x + ky = 5 will be inconsistent, is:

(a) k = 1                                (b) k = -1

(c) k ≠ -1                               (d) k ≠ 1

Solution:

(A) Answer (c) 4x – 3y = -4 and 4x + 3y = 20

Explanation: Given the cost of one ring game = ₹ x and cost of one balloon game = ₹ y.

According to the question,

4x – 3y = -4 and 4x + 3y = 20

(B) Answer (a) ₹ 2 and  ₹ 4

Explanation:From Solution (A)

4x – 3y = -4  ——(i)

4x + 3y = 20 ——- (ii)

Subtracting (i) to (ii)

(4x – 3y) – (4x + 3y) = -4 – 20

⇒ 4x – 3y – 4x – 3y = -24

⇒ – 6y = – 24

⇒ y = 4

Putting in equation (i)

4x – 3×4 = -4

⇒ 4x = -4 + 12

⇒ 4x = 8

⇒ x = 2

The cost of one ring game = ₹ 2

The cost of one balloon game = ₹ 4

(C) Answer (b) (-1, 0), (0, 4/3)

Explanation: The line represented by the equation 4x – 3y = -4 will intersect the x-axis for y = 0.

4x – 3×0 = -4

⇒ 4x = -4

⇒ x = -1

Therefore, point is (-1, 0)

The line will intersect the y-axis for x = 0.

So, -3y = -4

⇒ y = 4/3

Therefore the point is (0, 4/3)

(D) Answer (d) 12 sq. units

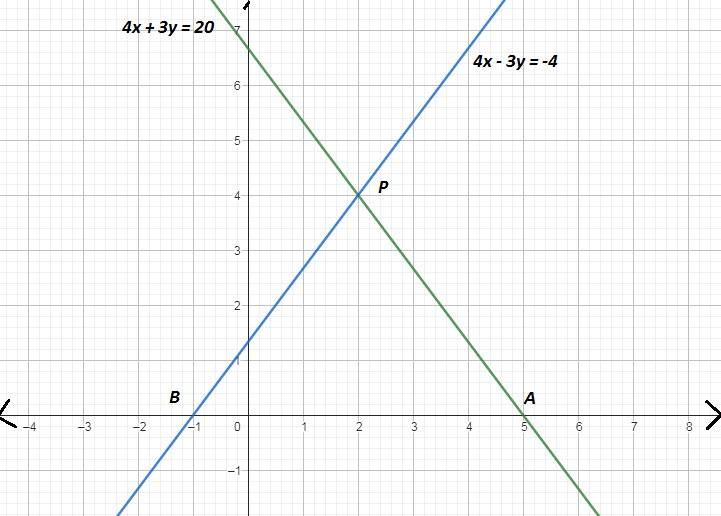
Class 10 Case based problem of Chapter 3 Pair of Linear eq 1

Area of triangle ΔABP = \frac{1}{2} × Base × Height

= 4\frac{1}{2} \times 4 \times 6

 = 12 sq. units

(E) Answer (b) k = -1

Explanation: For the pair of linear equation to be incosistent is

\dfrac{a_1}{a_2}=\dfrac{b_1}{b_2}\neq\dfrac{c_1}{c_2}

\Rightarrow \dfrac{-1}{1}=\dfrac{1}{k}\neq \dfrac{-1}{5}

\Rightarrow k \neq -1

 

The pictures are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures,their curve represents an efficient method of carrying load and so can be found in bridges and in architecture in a variety of forms.(Class 10 Case based problem of Chapter 2 Polynomials 3)
The pictures are few natural examples of parabolic shape which is represented by a quadratic

Class 10 Case based problem of Chapter 2 Polynomials 2

Class 10 Case based problem of Chapter 2 Polynomials 1

gmath.in

Leave a Comment