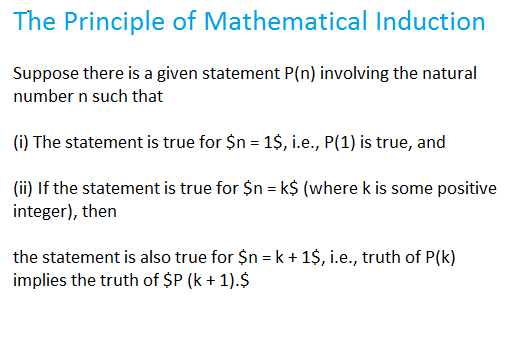
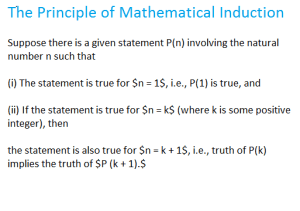
Chapter 4:(Principle Of Mathematical Induction)

Prove the following by using the principle of mathematical induction for all n ∈ N (Exercise 4.1 ncert math solution class 11)

Question 1:- $1+3+3^{2}+\ldots+3^{n-1}=\frac{\left(3^{n}-1\right)}{2}$

Solution: Let $P(n)$ be the given statement

$P(n): 1+3+3^{2}+\ldots+3^{n-1}=\frac{\left(3^{n}-1\right)}{2}$

For $n=1$ ,

$L.H.S.=1$

$R.H.S.=\frac{\left(3^{1}-1\right)}{2}=\frac{2}{2}=1 \text {, which is true. }$

Assume that $P(k)$ is true for some positive integer $k$ , such that

$P(k): 1+3+3^{2}+\ldots+3^{k-1}=\frac{\left(3^{k}-1\right)}{2}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$1+3+3^{2}+\ldots+3^{k-1}+3^k=\frac{\left(3^{k+1}-1\right)}{2}$

Taking L.H.S.

$\Rightarrow \left(1+3+3^{2}+\ldots+3^{k-1}\right)+3^{k}$

$\Rightarrow \frac{\left(3^{k}-1\right)}{2}+3^{k} \quad \text{From(1)}$

$\Rightarrow \frac{3^{k}-1+2 \times 3^{k}}{2}$

$\Rightarrow \frac{(1+2) 3^{k}-1}{2}$

$\Rightarrow \frac{3 \times 3^{k}-1}{2}$

$\Rightarrow \frac{3^{k+1}-1}{2}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true. Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 2:- $1^{3}+2^{3}+3^{3}+\ldots+n^{3}=\left[\frac{n(n+1)}{2}\right]^{2}$

Solution: Let $P(n)$ be the given statement.

$P(n): 1^{3}+2^{3}+3^{3}+\ldots+n^{3}=\left[\frac{n(n+1)}{2}\right]^{2}$

For $n=1$ ,

$L.H.S.= 1^3 = 1$

$R.H.S.=\left[\frac{1 \times 2}{2}\right]^{2}=[1]^{2}=1$

which is true for n= 1

Let $P(k)$ is true for some positive integer $k$

$P(k):1^{3}+2^{3}+3^{3}+\ldots+k^{3}=\left[\frac{k(k+1)}{2}\right]^{2}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$1^{3}+2^{3}+3^{3}+\ldots+k^3+(k+1)^{3}=\left[\frac{(k+1)(k+2)}{2}\right]^{2}$

Taking L.H.S.

$\left(1^{3}+2^{3}+3^{3}+\ldots+k^{3}\right)+(k+1)^{3}$

$\Rightarrow {\left[\frac{k(k+1)}{2}\right]^{2}+(k+1)^{3} \quad \ldots[\text { from }(1)] }$

$\Rightarrow \frac{k^{2}(k+1)^{2}}{4}+(k+1)^{3}$

$\Rightarrow \frac{k^{2}(k+1)^{2}+4(k+1)^{3}}{4}$

$\Rightarrow \frac{(k+1)^{2}\left[k^{2}+4(k+1)\right]}{4}$

$\Rightarrow \frac{(k+1)^{2}\left[k^{2}+4 k+4\right]}{4}$

$\Rightarrow \frac{(k+1)^{2}(k+2)^{2}}{4}$

$\Rightarrow {\left[\frac{(k+1)(k+2)}{2}\right]^{2} }$

${\left[\frac{(k+1)(k+1+1)}{2}\right]^{2} }$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 3: $1+\frac{1}{(1+2)}+\frac{1}{(1+2+3)}+\ldots+\frac{1}{(1+2+3+\ldots n)}=\frac{2 n}{(n+1)}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): 1+\frac{1}{(1+2)}+\frac{1}{(1+2+3)}+\ldots+\frac{1}{(1+2+3+\ldots n)}=\frac{2 n}{(n+1)}$

For $n=1$ ,

$L.H.S.= 1$

$R.H.S.=\frac{2 \times 1}{(1+1)}=\frac{2}{2}=1$ ,

which is true for n= 1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):1+\frac{1}{(1+2)}+\frac{1}{(1+2+3)}+\ldots+\frac{1}{(1+2+3+\ldots k)}=\frac{2 k}{(k+1)}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$1+\frac{1}{(1+2)}+\frac{1}{(1+2+3)}+\ldots+\frac{1}{(1+2+3+\ldots k)}+\frac{1}{(1+2+3+\ldots(k+1))}=\frac{2(k+1)}{(k+2)}$

Taking L.H.S.

$\Rightarrow\left[1+\frac{1}{(1+2)}+\frac{1}{(1+2+3)}+\ldots+\frac{1}{(1+2+3+\ldots k)}\right]+\frac{1}{(1+2+3+\ldots+k+(k+1))}$

$\Rightarrow \frac{2 k}{(k+1)}+\frac{1}{(1+2+3+\ldots+k+(k+1))} \quad \ldots[\text { from }(1)]$

Since $\left[ 1+2+3+\ldots+n=\frac{n(n+1)}{2}\right]$

$\Rightarrow \frac{2 k}{(k+1)}+\frac{2}{(k+1)(k+2)}$

$\Rightarrow \frac{2 k(k+2)+2}{(k+1)(k+2)}$

$\Rightarrow \frac{2\left(k^{2}+2 k+1\right)}{(k+1)(k+2)}$

$\Rightarrow \frac{2(k+1)^{2}}{(k+1)(k+2)}$

$\Rightarrow \frac{2(k+1)}{(k+2)}$

$\Rightarrow \frac{2(k+1)}{(k+1)+1}=R.H.S.$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 4: $1.2 .3+2.3 .4+\ldots+n(n+1)(n+2)=\frac{n(n+1)(n+2)(n+3)}{4}$

Solution: Let $P(n)$ be the given statement.

$P(n): 1.2 .3+2.3 .4+\ldots+n(n+1)(n+2)=\frac{n(n+1)(n+2)(n+3)}{4}$

For $n=1$ ,

$L.H.S.=1.2 .3=6$

$R.H.S.=\frac{1(1+1)(1+2)(1+3)}{4}=\frac{1.2 .3 .4}{4}=6$

which is true.

Assume that $P(k)$ is true for some positive integer $k$

$1.2 .3+2.3 .4+\ldots+k(k+1)(k+2)=\frac{k(k+1)(k+2)(k+3)}{4}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):1.2 .3+2.3 .4+\ldots+k(k+1)(k+2)+(k+1)[(k+2](k+3)=\frac{(k+1)(k+2)(k+3)(k+4)}{4}$

Taking L.H.S.

$1.2 .3+2.3 .4+\ldots+k(k+1)(k+2)+(k+1)(k+2)(k+3)$

$\Rightarrow \frac{k(k+1)(k+2)(k+3)}{4}+(k+1)(k+2)(k+3) \quad \ldots[\text { from }(1)]$

$\Rightarrow \frac{k(k+1)(k+2)(k+3)+4(k+1)(k+2)(k+3)}{4}$

$\Rightarrow \frac{(k+1)(k+2)(k+3)(k+4)}{4}$

$\Rightarrow \frac{(k+1)[(k+1)+1][(k+1)+2][(k+1)+3]}{4}$

$=R.H.S.$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 5: $1.3+2.3^{2}+3.3^{3}+\ldots+n .3^{n}=\frac{(2 n-1) 3^{n+1}+3}{4}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): 1.3+2.3^{2}+3.3^{3}+\ldots+n \cdot 3^{n}=\frac{(2 n-1) 3^{n+1}+3}{4}$

For $n=1$ ,

$L.H.S.=1.3=3$

$R.H.S.=\frac{(2 \times 1-1) 3^{1+1}+3}{4}=\frac{1.3^{2}+3}{4}=\frac{12}{4}=3$

which is true

Assume that $P(k)$ is true for some positive integer $k$

$1.3+2.3^{2}+3.3^{3}+\ldots+k \cdot 3^{k}=\frac{(2 k-1) 3^{k+1}+3}{4}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$1.3+2 \cdot 3^{2}+3 \cdot 3^{3}+\ldots+k \cdot 3^{k}+(k+1) \cdot 3^{k+1}=\frac{(2k+1) \cdot 3^{(k+2)}+3}{4}$

Taking L.H.S.

$1.3+2 \cdot 3^{2}+3 \cdot 3^{3}+\ldots+(k+1) \cdot 3^{k+1}$

$\Rightarrow {\left[1.3+2 \cdot 3^{2}+3 \cdot 3^{3}+\ldots+k \cdot 3^{k}\right]+(k+1) \cdot 3^{k+1} }$

$\Rightarrow \frac{(2 k-1) 3^{k+1}+3}{4}+(k+1) \cdot 3^{k+1} \quad \ldots[\text { from }(1)]$

$\Rightarrow \frac{(2 k-1) 3^{k+1}+3+4(k+1) \cdot 3^{k+1}}{4}$

$\Rightarrow \frac{3^{k+1}[(2 k-1)+4(k+1)]+3}{4}$

$\Rightarrow \frac{3^{k+1}[2 k-1+4 k+4]+3}{4}$

$\Rightarrow \frac{3^{k+1}[6 k+3]+3}{4}$

$\Rightarrow \frac{(2k+1) \cdot 3^{(k+1)+1}+3}{4}$

$=R.H.S.$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 6: $1.2+2.3+3.4+\ldots+n \cdot(n+1)=\left[\frac{n(n+1)(n+2)}{3}\right]$ .

Solution: Let $P(n)$ be the given statement. i.e.,

$P(n): 1.2+2.3+3.4+\ldots+n \cdot(n+1)=\left[\frac{n(n+1)(n+2)}{3}\right]$

For $n=1$ ,

$L.H.S. 1.2=2$

$R.H.S.=\left[\frac{1(1+1)(1+2)}{3}\right]=\frac{1.2 .3}{3}=2$

$L.H.S.=R.H.S.$

which is true for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k): 1.2+2.3+3.4+\ldots+k .(k+1)=\left[\frac{k(k+1)(k+2)}{3}\right]--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have
$P(k+1):1.2+2.3+3.4+\ldots+(k+1)(k+2)=\frac{(k+1)(k+2)(k+3)}{3}$
Taking L.H.S.

$1.2+2.3+3.4+\ldots+(k+1)(k+2)$

$\Rightarrow {[1.2+2.3+3.4+\ldots+k \cdot(k+1)]+(k+1)(k+2) }$

$\Rightarrow {\left[\frac{k(k+1)(k+2)}{3}\right]+(k+1)(k+2) \quad \ldots[\text { from }(1)] }$

$\Rightarrow \frac{k(k+1)(k+2)+3(k+1)(k+2)}{3}$

$\Rightarrow \frac{(k+1)(k+2)(k+3)}{3}$

$\Rightarrow \frac{(k+1)[(k+1)+1][(k+1)+2]}{3}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 7: $1.3+3.5+5.7+\ldots+(2 n-1)(2 n+1)=\frac{n\left(4 n^{2}+6 n-1\right)}{3}$ .

Solution: Let $P(n)$ be the given statement. For $n=1$ ,

$P(n): 1.3+3.5+5.7+\ldots+(2 n-1)(2 n+1)=\frac{n\left(4 n^{2}+6 n-1\right)}{3}$

$L.H.S.= 1.3=3$

$=\frac{1\left(4.1^{2}+6.1-1\right)}{3}=\frac{9}{3}=3$

Hence, $L.H.S.=R.H.S.$ for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):1.3+3.5+5.7+\ldots+(2 k-1)(2 k+1)=\frac{k\left(4 k^{2}+6 k-1\right)}{3}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):1.3+3.5+5.7+\ldots+[2(k+1)-1]+[2(k+1)+1]=\frac{(k+1)[4(k+1)^2+6(k+1)-1]}{3}$

Taking L.H.S.

$1.3+3.5+5.7+\ldots+[2(k+1)-1]+[2(k+1)+1]$

$\Rightarrow {1.3+3.5+5.7+\ldots+(2 k-1)(2 k+1)+(2 k+1)(2 k+3) }$

$\Rightarrow {\left[\frac{k\left(4 k^{2}+6 k-1\right)}{3}\right]+\left(4 k^{2}+8 k+3\right) \quad \ldots[\text { from }(1)] }$

$\Rightarrow \frac{k\left(4 k^{2}+6 k-1\right)+3\left(4 k^{2}+8 k+3\right)}{3}$

$\Rightarrow \frac{4 k^{3}+6 k^{2}-k+12 k^{2}+24 k+9}{3}$

$\Rightarrow \frac{4 k^{3}+18 k^{2}+23 k+9}{3}$

$\Rightarrow \frac{4 k^{3}+14 k^{2}+9 k+4 k^{2}+14 k+9}{3}$

$\Rightarrow \frac{\left(k k^{2}+14 k+9\right)+\left(4 k^{2}+14 k+9\right)}{3}$

$\Rightarrow \frac{(k+1)\left(4 k^{2}+14 k+9\right)}{3}$

$\Rightarrow \frac{(k+1)\left(4 k^{2}+8 k+4+6 k+6-1\right)}{3}$

$\Rightarrow \frac{(k+1)\left[4\left(k^{2}+2 k+2\right)+6(k+1)-1\right]}{3}$

$\Rightarrow \frac{(k+1)[4(k+1)^2+6(k+1)-1]}{3}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 8: $1.2+2.2^{2}+3.2^{3}+\ldots+n \cdot 2^{n}=(n-1) 2^{n+1}+2$ .

Solution: Let $P(n)$ be the given statement.

$P(n): 1.2+2.2^{2}+3.2^{3}+\ldots+n \cdot 2^{n}=(n-1) 2^{n+1}+2$

For $n=1$ ,

$L.H.S.= 1.2=2$

$=(1-1) 2^{1+1}+2=0+2=2$

$L.H.S.=R.H.S.$ for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):1.2+2.2^{2}+3.2^{3}+\ldots+k .2^{n}=(k-1) 2^{k+1}+2$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):1 \cdot 2+2 \cdot 2^{2}+3 \cdot 2^{3}+\ldots+(k+1) \cdot 2^{k+1}= {[(k+1)-1] \cdot 2^{(k+1)+1}+2 }$

Taking L.H.S.

$1 \cdot 2+2 \cdot 2^{2}+3 \cdot 2^{3}+\ldots+(k+1) \cdot 2^{k+1}$

$\Rightarrow {\left[1 \cdot 2+2 \cdot 2^{2}+3 \cdot 2^{3}+\ldots+k \cdot 2^{n}\right]+(k+1) \cdot 2^{k+1} }$

$\Rightarrow (k-1) 2^{k+1}+2+(k+1) \cdot 2^{k+1} \quad \ldots[\text { from }(1)]$

$\Rightarrow {[(k-1)+(k+1)] 2^{k+1}+2 }$

$\Rightarrow 2 k \cdot 2^{(k+1)}+2$

$\Rightarrow k \cdot 2^{(k+1)+1}+2$

$\Rightarrow {[(k+1)-1] \cdot 2^{(k+1)+1}+2 }$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$

Question 9: $\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{n}}=1-\frac{1}{2^{n}}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): \frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{n}}=1-\frac{1}{2^{n}}$

For $n=1$ ,

$L.H.S.=\frac{1}{2}$

$=1-\frac{1}{2^{1}}=1-\frac{1}{2}=\frac{1}{2}$

Hence, L.H.S. = R.H.S. for n= 1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{k}}=1-\frac{1}{2^{k}}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{k+1}}=1-\frac{1}{2^{k+1}}$

Taking L.H.S.

$\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{k+1}}$

$\Rightarrow {\left[\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\ldots+\frac{1}{2^{k}}\right]+\frac{1}{2^{k+1}} }$

$\Rightarrow 1-\frac{1}{2^{k}}+\frac{1}{2^{k+1}} \quad \ldots[\text { from (1) }]$

$\Rightarrow 1-\frac{1}{2^{k}}\left(1-\frac{1}{2}\right)$

$\Rightarrow 1-\frac{1}{2^{k}} \cdot \frac{1}{2}$

$\Rightarrow 1-\frac{1}{2^{k+1}}$

$=R.H.S.$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 10: $\frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{(3 n-1)(3 n+2)}=\frac{n}{(6 n+4)}$

Solution: Let $P(n)$ be the given statement.

$P(n): \frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{(3 n-1)(3 n+2)}=\frac{n}{(6 n+4)}$

For $n=1$ ,

$L.H.S.=\frac{1}{2.5}=\frac{1}{10}$

$R.H.S.=\frac{1}{(6.1+4)}=\frac{1}{10}$

L.H.S.=R.H.S. for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{(3 k-1)(3 k+2)}=\frac{k}{(6 k+4)}--(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$\frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{[3(k+1)-1][3(k+1)+2]}=\frac{(k+1)}{[6(k+1)+4]}$

Taking L.H.S.

$\frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{[3(k+1)-1][3(k+1)+2]}$

$\Rightarrow\left[\frac{1}{2.5}+\frac{1}{5.8}+\frac{1}{8.11}+\ldots+\frac{1}{(3 k-1)(3 k+2)}\right]+\frac{1}{(3 k+2)(3 k+5)}$

$\Rightarrow \frac{k}{(6 k+4)}+\frac{1}{(3 k+2)(3 k+5)} \quad \ldots[\text { from }(1)]$

$\Rightarrow \frac{k}{2(3 k+2)}+\frac{1}{(3 k+2)(3 k+5)}$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{k}{2}+\frac{1}{(3 k+5)}\right]$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{k(3 k+5)+2}{2(3 k+5)}\right]$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{3 k^{2}+5 k+2}{2(3 k+5)}\right]$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{3 k^{2}+3 k+2 k+2}{2(3 k+5)}\right]$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{3 k(k+1)+2(k+1)}{2(3 k+5)}\right]$

$\Rightarrow \frac{1}{(3 k+2)}\left[\frac{(k+1)(3 k+2)}{2(3 k+5)}\right]$

$\Rightarrow \frac{(k+1)}{(6 k+10)}$

$\Rightarrow \frac{(k+1)}{[(6 k+6)+4]}$

$\Rightarrow \frac{(k+1)}{[6(k+1)+4]}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 11: $\frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{n(n+1)(n+2)}=\frac{n(n+3)}{4(n+1)(n+2)}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): \frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{n(n+1)(n+2)}=\frac{n(n+3)}{4(n+1)(n+2)}$

For $n=1$ ,

$L.H.S.=\frac{1}{1.2 .3}=\frac{1}{6}$

$R.H.S.=\frac{1(1+3)}{4(1+1)(1+2)}=\frac{1.4}{4.2 .3}=\frac{1}{6}$

Hence L.H.S.=R.H.S. for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{k(k+1)(k+2)}=\frac{k(k+3)}{4(k+1)(k+2)}---(i)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):\frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{(k+1)[(k+1)+1][(k+1)+2]}=\frac{(k+1)[(k+1)+3]}{4[(k+1)+1][(k+1)+2]}$

Taking L.H.S.

$\frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{(k+1)[(k+1)+1][(k+1)+2]}$

$\Rightarrow\left[\frac{1}{1.2 .3}+\frac{1}{2.3 .4}+\frac{1}{3.4 .5}+\ldots+\frac{1}{k(k+1)(k+2)}\right]+\frac{1}{(k+1)(k+2)(k+3)}$

$\Rightarrow \frac{k(k+3)}{4(k+1)(k+2)}+\frac{1}{(k+1)(k+2)(k+3)} \quad \ldots[\text { from }(1)]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k(k+3)}{4}+\frac{1}{(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k(k+3)^{2}+4}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k\left(k^{2}+6 k+9\right)+4}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k^{3}+6 k^{2}+9 k+4}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k^{3}+2 k^{2}+k+4 k^{2}+8 k+4}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{k\left(k^{2}+2 k+1\right)+4\left(k^{2}+2 k+1\right)}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{(k+4)\left(k^{2}+2 k+1\right)}{4(k+3)}\right]$

$\Rightarrow \frac{1}{(k+1)(k+2)}\left[\frac{(k+4)(k+1)^{2}}{4(k+3)}\right]$

$\Rightarrow \frac{(k+1)(k+4)}{4(k+2)(k+3)}$

$\Rightarrow \frac{(k+1)[(k+1)+3]}{4[(k+1)+1][(k+1)+2]}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 12: $a+a r+a r^{2}+\ldots+a r^{n-1}=\frac{a\left(r^{n}-1\right)}{r-1}$ .

Solution: Let $P(n)$ be the given statement.

$(n): a+a r+a r^{2}+\ldots+a r^{n-1}=\frac{a\left(r^{n}-1\right)}{r-1}$

For $n=1$ ,

$L.H.S.= a$

$R.H.S.=\frac{a\left(r^{1}-1\right)}{r-1}=\frac{a(r-1)}{r-1}=a$
Hence, L.H.S.=R.H.S. for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k): a+a r+a r^{2}+\ldots+a r^{k-1}=\frac{a\left(r^{k}-1\right)}{r-1}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):a+a r+a r^{2}+\ldots+a r^{(k+1)-1}=\frac{a\left(r^{k+1}-1\right)}{r-1}$

Taking L.H.S.

$a+a r+a r^{2}+\ldots+a r^{(k+1)-1}$

$\Rightarrow {\left[a+a r+a r^{2}+\ldots+a r^{k-1}\right]+a r^{k} }$

$\Rightarrow \frac{a\left(r^{k}-1\right)}{r-1}+a r^{k} \quad \ldots[\text { from (1) ] }$

$\Rightarrow \frac{a\left(r^{k}-1\right)+a r^{k}(r-1)}{r-1}$

$\Rightarrow \frac{a r^{k}-a+a r^{k+1}-a r^{k}}{r-1}$

$\Rightarrow \frac{a r^{k+1}-a}{r-1}$

$\Rightarrow \frac{a\left(r^{k+1}-1\right)}{r-1}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 13 : $\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{(2 n+1)}{n^{2}}\right)=(n+1)^{2}$ .

Solution: Let $P(n)$ be the given statement.

$P(n):\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{(2 n+1)}{n^{2}}\right)=(n+1)^{2}$

For $n=1$ ,

$L.H.S.=\left(1+\frac{3}{1}\right)=4$

$R.H.S.=(1+1)^{2}=2^{2}=4$

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{(2 k+1)}{k^{2}}\right)=(k+1)^{2}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{[2(k+1)+1]}{(k+1)^{2}}\right)=(k+1+1)^{2}$

Taking L.H.S.

$\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{[2(k+1)+1]}{(k+1)^{2}}\right)$

$\Rightarrow {\left[\left(1+\frac{3}{1}\right)\left(1+\frac{5}{4}\right)\left(1+\frac{7}{9}\right) \ldots\left(1+\frac{(2 k+1)}{k^{2}}\right)\right]\left(1+\frac{(2 k+3)}{(k+1)^{2}}\right) }$

$\Rightarrow (k+1)^{2}\left(1+\frac{(2 k+3)}{(k+1)^{2}}\right) \quad \ldots[\text { from }(1)]$

$\Rightarrow (k+1)^{2}\left(\frac{(k+1)^{2}+(2 k+3)}{(k+1)^{2}}\right)$

$\Rightarrow (k+1)^{2}+(2 k+3)$

$\Rightarrow k^{2}+2 k+1+2 k+3$

$\Rightarrow k^{2}+4 k+4$

$\Rightarrow (k+2)^{2}$

$\Rightarrow (k+1+1)^{2}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 14 : $\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \ldots\left(1+\frac{1}{n}\right)=(n+1)$ .

Solution: Let $P(n)$ be the given statement.

$P(n):\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \ldots\left(1+\frac{1}{n}\right)=(n+1)$

For $n=1$ ,

$L.H.S.=\left(1+\frac{1}{1}\right)=2$

$=(1+1)=2$

Hence, L.H.S. = R.H.S. for n = 1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \ldots\left(1+\frac{1}{k}\right)=(k+1)$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \cdots\left(1+\frac{1}{k+1}\right)={[(k+1)+1] }$

Taking L.H.S.

$\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \cdots\left(1+\frac{1}{k+1}\right)$

$\Rightarrow {\left[\left(1+\frac{1}{1}\right)\left(1+\frac{1}{2}\right)\left(1+\frac{1}{3}\right) \ldots\left(1+\frac{1}{k}\right)\right]\left(1+\frac{1}{k+1}\right) }$

$\Rightarrow (k+1)\left(1+\frac{1}{k+1}\right)$

$\Rightarrow (k+1)\left(\frac{k+1+1}{k+1}\right)$

$\Rightarrow {[(k+1)+1] }$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 15: $1^{2}+3^{2}+5^{2}+\ldots+(2 n-1)^{2}=\frac{n(2 n-1)(2 n+1)}{3}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): 1^{2}+3^{2}+5^{2}+\ldots+(2 n-1)^{2}=\frac{n(2 n-1)(2 n+1)}{3}$

For $n=1$ ,

$L.H.S.=1^{2}=1$

$=\frac{1(2.1-1)(2.1+1)}{3}=\frac{1.1 .3}{3}=1$

Hence, L.H.S. = R.H.S. for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):1^{2}+3^{2}+5^{2}+\ldots+(2 k-1)^{2}=\frac{k(2 k-1)(2 k+1)}{3}--(i)$

We will now prove that $P(k+1)$ is also true. Now, we have

$P(k+1):1^{2}+3^{2}+5^{2}+\ldots+[2(k+1)-1]^{2}=\frac{(k+1)[2(k+1)-1][2(k+1)+1]}{3}$

Taking L.H.S.

$1^{2}+3^{2}+5^{2}+\ldots+[2(k+1)-1]^{2}$

$\Rightarrow\left[1^{2}+3^{2}+5^{2}+\ldots+(2 k-1)^{2}\right]+(2 k+1)^{2}$

$\Rightarrow \frac{k(2 k-1)(2 k+1)}{3}+(2 k+1)^{2} \quad \ldots[\text { from (1) }$

$\Rightarrow \frac{k(2 k-1)(2 k+1)+3(2 k+1)^{2}}{3}$

$\Rightarrow \frac{(2 k+1)[k(2 k-1)+3(2 k+1)]}{3}$

$\Rightarrow \frac{(2 k+1)\left[2 k^{2}-k+6 k+3\right]}{3}$

$\Rightarrow \frac{(2 k+1)\left[2 k^{2}+5 k+3\right]}{3}$

$\Rightarrow \frac{(2 k+1)\left[2 k^{2}+2 k+3 k+3\right]}{3}$

$\Rightarrow \frac{(2 k+1)[2 k(k+1)+3(k+1)]}{3}$

$\Rightarrow \frac{(2 k+1)(k+1)(2 k+3)}{3}$

$\Rightarrow \frac{(k+1)[2(k+1)-1][2(k+1)+1]}{3}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 16: $\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{(3 n-2)(3 n+1)}=\frac{n}{(3 n+1)}$ .

Solution: Let $P(n)$ be the given statement.

$P(n):\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{(3 n-2)(3 n+1)}=\frac{n}{(3 n+1)}$

For $n=1$ ,

$L.H.S.=\frac{1}{1.4}=\frac{1}{4}$

$=\frac{1}{(3.1+1)}=\frac{1}{4}$

Hence, L.H.S.=R.H.S., for n = 1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{(3 k-2)(3 k+1)}=\frac{k}{(3 k+1)}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(K+1):\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{[3(k+1)-2][3(k+1)+1]}\frac{(k+1)}{[3(k+1)+1]}$

Taking L.H.S.

$\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{[3(k+1)-2][3(k+1)+1]}$

$\Rightarrow {\left[\frac{1}{1.4}+\frac{1}{4.7}+\frac{1}{7.10}+\ldots+\frac{1}{(3 k-2)(3 k+1)}\right]+\frac{1}{(3 k+1)(3 k+4)} }$

$\Rightarrow \frac{k}{(3 k+1)}+\frac{1}{(3 k+1)(3 k+4)} \quad \ldots[\text { from }(1)]$

$\Rightarrow \frac{k(3 k+4)+1}{(3 k+1)(3 k+4)}$

$\Rightarrow \frac{3 k^{2}+4 k+1}{(3 k+1)(3 k+4)}$

$\Rightarrow \frac{3 k^{2}+3 k+k+1}{(3 k+1)(3 k+4)}$

$\Rightarrow \frac{3 k(k+1)+(k+1)}{(3 k+1)(3 k+4)}$

$\Rightarrow \frac{(3 k+1)(k+1)}{(3 k+1)(3 k+4)}$

$\Rightarrow \frac{(k+1)}{[3(k+1)+1]}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 17: $\frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{(2 n+1)(2 n+3)}=\frac{n}{3(2 n+3)}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): \frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{(2 n+1)(2 n+3)}=\frac{n}{3(2 n+3)}$

For $n=1$ ,

$L.H.S.=\frac{1}{3.5}=\frac{1}{15}$

$=\frac{1}{3(2.1+3)}=\frac{1}{3.5}=\frac{1}{15}$

Hence, L.H.S. = R.H.S. for n=1

Assume that $P(k)$ is true for some positive integer $k$

$P(k):\frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{(2 k+1)(2 k+3)}=\frac{k}{3(2 k+3)}$

We will now prove that $P(k+1)$ is also true.

Now, we have

$P(k+1):\frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{[2(k+1)+1][2(k+1)+3]}=\frac{(k+1)}{3[2(k+1)+3]}$

Taking L.H.S.

$\frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{[2(k+1)+1][2(k+1)+3]}$

$\Rightarrow {\left[\frac{1}{3.5}+\frac{1}{5.7}+\frac{1}{7.9}+\ldots+\frac{1}{(2 k+1)(2 k+3)}\right]+\frac{1}{(2 k+3)(2 k+5)} }$

$\Rightarrow \frac{k}{3(2 k+3)}+\frac{1}{(2 k+3)(2 k+5)}$

$\Rightarrow \frac{k(2 k+5)+3}{3(2 k+3)(2 k+5)}$

$\Rightarrow \frac{2 k^{2}+5 k+3}{3(2 k+3)(2 k+5)}$

$\Rightarrow \frac{2 k^{2}+2 k+3 k+3}{3(2 k+3)(2 k+5)}$

$\Rightarrow \frac{2 k(k+1)+3(k+1)}{3(2 k+3)(2 k+5)}$

$\Rightarrow \frac{(2 k+3)(k+1)}{3(2 k+3)(2 k+5)}$

$\Rightarrow \frac{(k+1)}{3(2 k+5)}$

$\Rightarrow \frac{(k+1)}{3[2(k+1)+3]}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 18 : $1+2+3+\ldots+n<\frac{1}{8}(2 n+1)^{2}$ .

Solution: Let $P(n)$ be the given statement.

$P(n): 1+2+3+\ldots+n<\frac{1}{8}(2 n+1)^{2}$

For $n=1$ ,

Since,

$P(1): 1<\frac{1}{8}(2.1+1)^{2}$

$\Rightarrow 1<\frac{9}{8}=1 \frac{1}{8}$

Hence, $P(1)$ is true

Assume that $P(k)$ is true for some positive integer $k$

$P(1):1+2+3+\ldots+k<\frac{1}{8}(2 k+1)^{2}--(i)$

We will now prove that $P(k+1)$ is true whenever $P(k)$ is true Now, we have

$1+2+3+\ldots+k <\frac{1}{8}(2 k+1)^{2} \quad[\text { from (1)] }$

Adding both side by $(k+1)$

$1+2+3+\ldots+k+(k+1) <\frac{1}{8}(2 k+1)^{2}+(k+1)$

$\Rightarrow 1+2+3+\ldots+k+(k+1)<\frac{1}{8}\left[(2 k+1)^{2}+8(k+1)\right]$

$\Rightarrow 1+2+3+\ldots+k+(k+1)<\frac{1}{8}\left[4 k^{2}+4 k+1+8 k+8\right]$

$\Rightarrow 1+2+3+\ldots+k+(k+1)<\frac{1}{8}\left[4 k^{2}+12 k+9\right]$

$\Rightarrow 1+2+3+\ldots+k+(k+1)<\frac{1}{8}[2 k+3]^{2}$

$\Rightarrow 1+2+3+\ldots+k+(k+1)<\frac{1}{8}[2(k+1)+1]^{2}$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 19: $n(n+1)(n+5)$ is a multiple of 3.

Solution: Let the statement be P(n)

$P(n): n(n+1)(n+5) \text { is a multiple of } 3 .$

For $n= 1$

$P(1): 1(1+1)(1+5)=1.2 .6=12 \text { which is a multiple of } 3 .$

Thus $P(n)$ is true for $n=1$

Let $P(k)$ be true for some natural number $k$ ,

$P(k): k(k+1)(k+5) \text { is a multiple of } 3 \text {. }$

Hence

$k(k+1)(k+5)=3 a$ where $a \in N$ .

$\Rightarrow k(k^2+6k+5)=3a$

$\Rightarrow k^3+6k^2+5k = 3a$

$\Rightarrow k^3 = 3a-6k^2-5k--(i)$

Now, we will prove that $P(k+1)$ is true

$P(k+1):(k+1)[(k+1)+1][(k+1)+5]$

$\Rightarrow (k+1)(k+2)(k+6)$

$\Rightarrow (k+1)(k^2+8k+12)$

$\Rightarrow k^3+8k^2+12k+k^2+8k+12$

$\Rightarrow (3a-6k^2-5k)+9k^2+20k+12\quad \text{ From (i) }$

$\Rightarrow 3a-6k^2-5k+9k^2+20k+12$

$\Rightarrow 3a+3k^2+15k+12$

$\Rightarrow 3(a+k^2+5k+4) \text { is a multiple of } 3 .$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 20: $10^{2 n-1}+1$ is divisible by 11 .

Solution: Let the statement be P(n)

$P(n): 10^{2 n-1}+1 \text { is divisible by } 11 \text {. }$

For $n = 1$

$P(1): 10^{2.1-1}+1=10+1=11 \text { which is divisible by } 11 \text {. }$

Thus $P(n)$ is true for $n=1$

Let $P(k)$ be true for some natural number $k$ ,

$P(k): 10^{2 k-1}+1 \text { is divisible by } 11 \text {. }$

We can write

$10^{2 k-1}+1=11 a \quad \text { where } a \in N .$

$\Rightarrow 10^{2k-1}=11a-1--(i)$

Now, we will prove that $P(k+1)$ is true

Now,

$P(k+1):10^{2(k+1)-1}+1$

$\Rightarrow 10^{2 k+1}+1$

$\Rightarrow 10^{2}\left(10^{2 k-1}\right)+1$

$\Rightarrow 100(11a-1)+1\quad \text{ From (i) }$

$\Rightarrow 1100a-100+1$

$\Rightarrow 1100a-99$

$\Rightarrow 11(100a-9)\quad \text{ is divisible by 11 }$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 21: $x^{2 n}-y^{2 n}$ is divisible by $x+y$ .

Solution: Let the statement be P(n)

$P(n): x^{2 n}-y^{2 n} \text { is divisible by } x+y .$

For $n=1$

$P(1): x^{2.1}-y^{2.1}$

$=x^{2}-y^{2}=(x+y)(x-y) \text { which is divisible by } x+y \text {. }$

Thus $P(n)$ is true for $n=1$

Let $P(k)$ be true for some natural number $k$ ,

$P(k): x^{2 k}-y^{2 k} \text { is divisible by } x+y \text {. }$

We can write it as

$x^{2 k}-y^{2 k}=a(x+y)\text { where } a \in N .$

$\Rightarrow x^{2k}=a(x+y)+y^{2k}--(i)$

Now, we will prove that $P(k+1)$ is true

Now,

$P(k+1):x^{2(k+1)}-y^{2(k+1)}$

$\Rightarrow x^{2 k+2}-y^{2 k+2}$

$\Rightarrow x^{2}\left(x^{2 k}\right)-y^{2}\left(y^{2 k}\right)$

$\Rightarrow x^2[a(x+y)+y^{2k}]-y^{2}\left(y^{2 k}\right)$

$\Rightarrow ax^2(x+y)+x^2y^{2k}-y^2y^{2k}$

$\Rightarrow ax^2(x+y)+y^{2k}(x^2-y^2)$

$\Rightarrow ax^2(x+y)+y^{2k}(x-y)(x+y)$

$\Rightarrow (x+y)\left[a x^{2}+(x-y) y^{2 k}\right] \text { is divisible by } x+y .$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 22: $3^{2 n+2}-8 n-9$ is divisible by 8 .

Solution: Let the statement be P(n)

$P(n): 3^{2 n+2}-8 n-9 \text { is divisible by } 8 .$

For $n=1$

$P(1): 3^{2.1+2}-8.1-9$

$=3^{4}-8-9=81-17=64 \text { which is divisible by } 8 \text {. }$

Thus $P(n)$ is true for $n=1$

Let $P(k)$ be true for some natural number $k$ ,

$P(k): 3^{2 k+2}-8 k-9 \text { is divisible by } 8 \text {. }$

$3^{2 k+2}-8 k-9=8 a(Let)$

$\Rightarrow 3^{2k+2}=8a+8k+9--(i)$

where $a \in N$ .

Now, we will prove that $P(k+1)$ is true .

Now,

$P(k+1):3^{2(k+1)+2}-8(k+1)-9$

$\Rightarrow 3^{2 k+4}-8 k-8-9$

$\Rightarrow 3^{2} \cdot 3^{2 k+2}-8 k-17$

$\Rightarrow 9(8a+8k+9)-8k-17\quad \text{ From (i) }$

$\Rightarrow 72a+72k+81-8k-17$

$\Rightarrow 72a+64k+64$

$\Rightarrow 8(9a+8k+8) \text { is divisible by } 8 \text {. }$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 23: $41^{n}-14^{n}$ is a multiple of 27.

Solution: Let the statement be P(n)

$P(n): 41^{n}-14^{n} \text { is a multiple of } 27 .$

$P(1): 41^{1}-14^{1}$

$=41-14=27 \text { which is a multiple of } 27 .$

Thus $P(n)$ is true for $n=1$

Let $P(k)$ be true for some natural number $k$ ,

$P(k): 41^{k}-14^{k} \text { is a multiple of } 27 \text {. }$

We can write

$41^{k}-14^{k}=27 a\text { where } a \in N .$

$\Rightarrow 41^k = 27a +14^k$

Now, we will prove that $P(k+1)$ is true

Now,

$41^{k+1}-14^{k+1}$

$\Rightarrow 41.41^{k}-14.14^{k}$

$\Rightarrow 41(27a+14^k)-14.14^k$

$\Rightarrow 41\times 27a+41.14^k-14.14^k$

$\Rightarrow 41\times 27a+14^k(41-14)$

$\Rightarrow 41\times 27a+14^k\times 27$

$\Rightarrow 27(41a+14^k)\text { is a multiple of } 27$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

Question 24 : $(2 n+7)<(n+3)^{2}$

Solution:Let $P(n)$ be the given statement.

$P(n):(2 n+7)<(n+3)^{2}$

We note that $P(n)$ is true for $n=1$ ,

$P(1):(2.1+7)=9<(1+3)^{2}=16$

Assume that $P(k)$ is true for some positive integer $k$

$P(k):2 k+7<(k+3)^{2}--(i)$

We will now prove that $P(k+1)$ is true,

Adding 2 both side in eq (i)

$(2 k+7)+2 <(k+3)^{2}+2$

$\Rightarrow (2 k+7)+2<k^{2}+6 k+9+2$

$\Rightarrow (2 k+7)+2<k^{2}+6 k+11$

Adding $2k+5 \text{ in R.H.S. }$

$\Rightarrow 2(k+1)+7<k^2+6k+11+2k+5$

$\Rightarrow 2(k+1)+7<k^2+8k+16$

$\Rightarrow 2(k+1)+7<(k+4)^2$

Thus $P(k+1)$ is true, whenever $P(k)$ is true.

Hence, from the principle of mathematical induction, the statement $P(n)$ is true for all natural numbers i.e., $n \in N$ .

gmath.in

Chapter 4:(Principle Of Mathematical Induction)

Leave a Comment Cancel reply