Case study Chapter 6 (Application of Derivative)

Case study 2:- Read the following and answer the question.(Case study application of derivative 2)

Dr. Ritam residing to Delhi went to see an apartment of 3 BHK in Noida. The window of the house was in the form of a rectangle surmounted by a semicircular opening having a perimeter of the window 10 m as shown in figure.

(i) If x and y represents the length and breadth of the recangular region, then the relation between the variable is

(a) $x+y+\frac{x}{2}=10$ (b) $x+2y+\frac{x}{2}=10$

(ii) The area of the window (A) expressed as a function of x is

(a) $A = x - \frac{\pi x^3}{8}-\frac{x^}{2}$ (b) $A=5x-\frac{x^2}{2}-\frac{\pi x^2}{8}$

(iii) Dr. Ritam is interested in maximising the area of the whole window. For this to happen the value of x should be

(a) 20/π (b) 20/(4-π)

(iv) For maximum value of A, the breadth of rectangular part of window is

(a) 20/(4+π) (b) 20/π

(v) The maximum area of window is

(a) $\frac{10}{(4+\pi)^2}$ sq.m (b) $\frac{10\pi}{(4+\pi)^2}$ sq.m

Solution: (i) Answer (c)

Since perimeter of window = x + y + y + perimeter of semicircle

[Since radius of semicircle is x/2]

$= x +2y+\frac{1}{2}\times 2\pi\times \frac{x}{2}$

$= x+2y+\frac{\pi x}{2}$

(ii) answer (b)

Since,

$A = x\times y +\frac{1}{2}\pi(\frac{x}{2})^2$

$= x.y+\frac{\pi x^2}{8}$

$\left[10 =x+2y+\frac{\pi x}{2} \Rightarrow y = (5-\frac{x}{2}-\frac{\pi x}{4})\right]$

$= x\left(5-\frac{x}{2}-\frac{\pi x}{4}\right)+\frac{\pi x^2}{8}$

$= 5x-\frac{x^2}{2}-\frac{\pi x^2}{4}+\frac{\pi x^2}{8}$

$= 5x-\frac{x^2}{2}-\frac{\pi x^2}{8}$

(iii) Answer (d)

For maximum value of A

$\frac{dA}{dx}=0$

$\Rightarrow 5-x-\frac{\pi x}{4}=0 \Rightarrow x + \frac{\pi x}{4}=5$

$\Rightarrow 4x + \pi x = 20 \Rightarrow x(\pi+x) = 20$

$\Rightarrow x = \frac{20}{4+\pi}$

(iv) Answer (c)

Since, $y=5-\frac{x}{2}-\frac{\pi x}{4}$

$= 5-\left(\frac{2x+\pi x}{4}\right) = 5-x.\left(\frac{2+\pi}{4}\right)$

$= 5-\frac{20}{4+\pi}.\frac{2+\pi}{4}$

$= \frac{20(4+\pi)-20(2+\pi)}{4(4+\pi)}$

$= \frac{80+20\pi-40-20\pi}{4(4+\pi)}$

$= \frac{40}{4(4+\pi)}=\frac{10}{4+\pi}$

(v) Answer (d)

Area of window = ar(rectangular part) + ar( semi circular part)

$= \frac{20}{4+\pi}.\frac{10}{4+\p}+\frac{1}{2}.\pi(\frac{x}{2})^2$

$= \frac{200}{(4+\pi)^2}+\frac{\pi x^2}{8}$

$= \frac{200}{(4+\pi)^2}+\frac{\pi}{8}\left(\frac{20}{4+\pi}\right)^2$

$= \frac{200}{(4+\pi)^2}+\frac{400\pi}{8(4+\pi)^2}=\frac{200+50\pi}{(4+\pi)^2}$

——————————————————————–

Some other Case study problem

Case study 1: Read the following and answer the question(Case study application of derivative 1 )
A recangular hall is to be developed for a meeting of farmers in an agriculture college to aware them for new technique in cultivation. It is given that the floor has a fixed perimeter P as shown below.

Solution: For solution click here

Case study 3:- Read the following and answer the question:(Case study application of derivative 3)

On the request of villagers, a construction agency designs a tank with the help of an architect. Tank consist of rectangular base with recangular sides, open at the top so that its depth is 2 m and volume is 8 cubic meter as shown below:

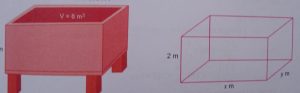
Solution: For solution click here

Case study 4:- Read the following and answer the question:(Case study application of derivative 4 )

These days chinese and Indian troops are engaged in aggressive melee,face-off skirmishes at location near the disputed Pangong Lake in Ladakh.

One day a helicopterof enemy is flying along the curve represented by y = x² + 7. A soldier placed at (3, 7) wants to shoot down the helicopter when it is nearest to him.

Solution: For solution click here

Case study 5:– Indian Railways is the largest rail network in Asia and world’s second largest. No doubt huge amount of money of Indian government paid as fuel cost. Decreasing fuel cost can increase railway profit and hence will improve Indian economy.(Case study application of derivative 5 )

Case study application of derivative 5 — Indian Railways is the largest rail network in Asia and world’s second largest. No doubt

This is the fact that the fuel cost for running a train is proportional to the square of the speed generated in km per hour. The fuel cost is ₹ 48 per hour at speed 16 km per hour and fixed charges amount to ₹ 1200 per hour.

Solution: For solution click here

—————————————————————-