Show that of all the rectangles inscribed in a given fixed circle

by

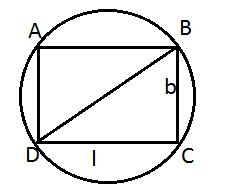
Question 3:- Show that of all the rectangles inscribed in a given fixed circle, the square has maximum area.

Solution: LetPQRS be the rectangle inscribed in a given circle with centre \mathrm{O} and radius a.

Let x and y be the length and breadth of the rectangle, that is, x>0 and y>0
In right angled  ΔPQR, using Pythagoras theorem,

class 12 maths ex 6.5 ncert solution

\mathrm{PQ}^2+\mathrm{QR}^2=\mathrm{PR}^2

x^2+y^2=(2 a)^2

y^2=4 a^2-x^2

y=\sqrt{4 a^2-x^2}–(i)

Let \mathrm{A} be the area of the rectangle,

then \mathrm{A}=\mathrm{xy}=x \sqrt{4 a^2-x^2}

Squaring both side

A^2 = S(let) =x^2(4a^2-x^2)

Differentiating with respect to x

\frac{dS}{dx} = x^2(0-2x)+(4a^2-x^2).2x

= 2x(-x^2+4a^2-x^2)

= 2x(4a^2-2x^2)—–(ii)

For max and minima \frac{dS}{dx} =0

2x(4a^2-2x^2)=0

\Rightarrow x = 0 or 2x^2 = 4a^2

\Rightarrow x = 0 or x =\sqrt{2}a

Again differentiate with respect to x of (ii)

\frac{d^2S}{dx^2 } = 2x(-4x)+(4a^2-2x^2)

= -8x^2+4a^2-2x^2

= 4a^2-10x^2

At x = \sqrt{2}a

\frac{d^2S}{dx^2} = 4a^2 -10\times 2a^2

= 4a^2-20a^2=-16a^2<0

Hence the area of the square is max when x=\sqrt{2}a

From eq (i)

y=\sqrt{4 a^2-2a^2}

\Rightarrow y= 2a^2

x = y =\sqrt{2}

Hence area of inscribed rectangle is maximum when it is a square

Question 1:- A square piece of tin of side 18 cm is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?

Solution:- For solution Click Here

Question 2:- A rectangula sheet of tin 45 cm by 24 cmis to be made into a box without top, by cutting of square from each corner and folding up the flaps. What should be the side of square to be cutt of so that the volume of the box is maximum?

Solution:- For solution Click here

Leave a Comment