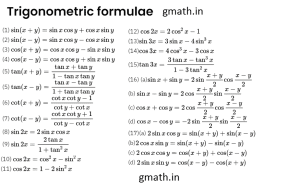
EXERCISE 3.3(Trigonometric Function)

Prove that:(Ex 3.3 Trigonomety ncert maths solution class 11)

Question 1: $\sin ^2 \frac{\pi}{6}+\cos ^2 \frac{\pi}{3}-\tan ^2 \frac{\pi}{4}=-\frac{1}{2}$

Solution: L.H.S. $=\sin ^2 \frac{\pi}{6}+\cos ^2 \frac{\pi}{3}-\tan ^2 \frac{\pi}{4}$

$=\left(\frac{1}{2}\right)^2+\left(\frac{1}{2}\right)^2-(1)^2$

$=1 / 4+1 / 4-1$

$=\frac{1+1-4}{4}=-\frac{2}{4}$

$=-1 / 2 =\text { RHS }$

Question 2: $2 \sin ^2 \frac{\pi}{6}+\operatorname{cosec}^2 \frac{7 \pi}{6} \cos ^2 \frac{\pi}{3}=\frac{3}{2}$

Solution: $\text { L.H.S. }=2 \sin ^2 \frac{\pi}{6}+\operatorname{cosec}^2 \frac{7 \pi}{6} \cos ^2 \frac{\pi}{3}$

$=2\left(\frac{1}{2}\right)^2+\operatorname{cosec}^2\left(\pi+\frac{\pi}{6}\right)\left(\frac{1}{2}\right)^2$

$=2 \times \frac{1}{4}+\left(\operatorname{cosec^2} \frac{\pi}{6}\right)\left(\frac{1}{4}\right)$

$=\frac{1}{2}+(-2)^2\left(\frac{1}{4}\right)$

$=1 / 2+4 / 4$

$=1 / 2+1$

$=3 / 2=\text { RHS }$

Question 3: $\cot ^2 \frac{\pi}{6}+\operatorname{cosec} \frac{5 \pi}{6}+3 \tan ^2 \frac{\pi}{6}=6$

Solution: L.H.S. $=\cot ^2 \frac{\pi}{6}+\operatorname{cosec} \frac{5 \pi}{6}+3 \tan ^2 \frac{\pi}{6}$

$=(\sqrt{3})^2+\operatorname{cosec}\left(\pi-\frac{\pi}{6}\right)+3\left(\frac{1}{\sqrt{3}}\right)^2$

$=3+\operatorname{cosec} \frac{\pi}{6}+3 \times \frac{1}{3}$

$=3+2+1$

$=6 =\text { RHS }$

Question 4: $2 \sin ^2 \frac{3 \pi}{4}+2 \cos ^2 \frac{\pi}{4}+2 \sec ^2 \frac{\pi}{3}=10$

Solution: L.H.S $=2 \sin ^2 \frac{3 \pi}{4}+2 \cos ^2 \frac{\pi}{4}+2 \sec ^2 \frac{\pi}{3}$

$=2\left\{\sin \left(\pi-\frac{\pi}{4}\right)\right\}^2+2\left(\frac{1}{\sqrt{2}}\right)^2+2(2)^2$

$=2\left\{\sin \frac{\pi}{4}\right\}^2+2 \times \frac{1}{2}+8$

$=2\left(\frac{1}{\sqrt{2}}\right)^2+1+8$

$=1+1+8$

$=10 =\text { RHS }$

Question 5: Find the value of:

(i) $\sin 75^{\circ}$

(ii) $\tan 15^{\circ}$

Solution: (i) $\sin 75^{\circ}$

$=\sin \left(45^{\circ}+30^{\circ}\right)$

Using the formula

$[\sin (x+y)=\sin x \cos y+\cos x \sin y]$

$=\sin 45^{\circ} \cos 30^{\circ}+\cos 45^{\circ} \sin 30^{\circ}$

$=\left(\frac{1}{\sqrt{2}}\right)\left(\frac{\sqrt{3}}{2}\right)+\left(\frac{1}{\sqrt{2}}\right)\left(\frac{1}{2}\right)$

$=\frac{\sqrt{3}}{2 \sqrt{2}}+\frac{1}{2 \sqrt{2}}$

$=\frac{\sqrt{3}+1}{2 \sqrt{2}}$

(ii) $\tan 15^{\circ}$

$=\tan \left(45^{\circ}-30^{\circ}\right)$

Using formula

$\tan (x-y)=\frac{\tan x-\tan y}{1+\tan x \tan y}$

$=\frac{\tan 45^{\circ}-\tan 30^{\circ}}{1+\tan 45^{\circ} \tan 30^{\circ}}$

Substituting the values

$=\frac{1-\frac{1}{\sqrt{3}}}{1+1\left(\frac{1}{\sqrt{3}}\right)}=\frac{\frac{\sqrt{3}-1}{\sqrt{3}}}{\frac{\sqrt{3}+1}{\sqrt{3}}}$

$=\frac{\sqrt{3}-1}{\sqrt{3}+1}$

Multiply by $\sqrt{3}-1$ in $N^r$ and $D^r$

$=\frac{\sqrt{3}-1}{\sqrt{3}+1}\times \frac{\sqrt{3}-1}{\sqrt{3}-1}$

$=\frac{(\sqrt{3}-1)^2}{(\sqrt{3}+1)(\sqrt{3}-1)}$

$=\frac{3+1-2 \sqrt{3}}{(\sqrt{3})^2-(1)^2}$

$=\frac{4-2 \sqrt{3}}{3-1}=\frac{2(2-\sqrt{3})}{2}$

$=2-\sqrt{3}$

Prove the following:

Question 6: $\cos \left(\frac{\pi}{4}-x\right) \cos \left(\frac{\pi}{4}-y\right)-\sin \left(\frac{\pi}{4}-x\right) \sin \left(\frac{\pi}{4}-y\right)=\sin (x+y)$

Solution: $LHS =\cos \left(\frac{\pi}{4}-x\right) \cos \left(\frac{\pi}{4}-y\right)-\sin \left(\frac{\pi}{4}-x\right) \sin \left(\frac{\pi}{4}-y\right)$

We know the formula

$\cos(x+y)=\cos x\cos y-\sin x\sin y$

Now,

$= \cos(\frac{\pi}{4}-x+\frac{\pi}{4}-y)$

$=\cos(\frac{\pi}{2}-(x+y))$

$=\sin(x+y)=RHS$

Question 7: $\frac{\tan \left(\frac{\pi}{4}+x\right)}{\tan \left(\frac{\pi}{4}-x\right)}=\left(\frac{1+\tan x}{1-\tan x}\right)^2$

Solution: $\text { .L.H.S. }=\frac{\tan \left(\frac{\pi}{4}+x\right)}{\tan \left(\frac{\pi}{4}-x\right)}$

By using the formula

$\tan (A+B)=\frac{\tan A+\tan B}{1-\tan A \tan B} \text { and } \tan (A-B)=\frac{\tan A-\tan B}{1+\tan A \tan B}$

So we get

$=\frac{\left(\frac{\tan \frac{\pi}{4}+\tan x}{1-\tan \frac{\pi}{4} \tan x}\right)}{\left(\frac{\tan \frac{\pi}{4}-\tan x}{1+\tan \frac{\pi}{4} \tan x}\right)}$

$= \frac{\left(\frac{1+\tan x}{1-\tan x}\right)}{\left(\frac{1-\tan x}{1+\tan x}\right)}$

$= \left(\frac{1+\tan x}{1-\tan x}\right)^2= \text { RHS }$

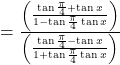
Question 8: $\frac{\cos (\pi+x) \cos (-x)}{\sin (\pi-x) \cos \left(\frac{\pi}{2}+x\right)}=\cot ^2 x$

Solution: $\text { L.H.S. }=\frac{\cos (\pi+x) \cos (-x)}{\sin (\pi-x) \cos \left(\frac{\pi}{2}+x\right)}$

$=\frac{(-\cos x)(\cos x)}{(\sin x)(-\sin x)}$

$=\frac{-\cos ^2 x}{-\sin ^2 x}$

$=\cot ^2 \mathrm{x} ={RHS}$

Question 9: $\cos \left(\frac{3 \pi}{2}+x\right) \cos (2 \pi+x)\left[\cot \left(\frac{3 \pi}{2}-x\right)+\cot (2 \pi+x)\right]=1$

Solution: $\text { L.H.S. }=\cos \left(\frac{3 \pi}{2}+x\right) \cos (2 \pi+x)\left[\cot \left(\frac{3 \pi}{2}-x\right)+\cot (2 \pi+x)\right]$

$=\sin \mathrm{x} \cos \mathrm{x}(\tan \mathrm{x}+\cot \mathrm{x})$

$=\sin x.\cos x(\frac{\sin x}{\cos x}+\frac{\cos x}{\sin x})$

$=\sin x\cos x(\frac{\sin^2x+\cos^2 x}{\sin x\cos x})$

$=1=RHS$

Question 10: $\sin(n+1)x\sin(n+2)x+\cos(n+1)x\cos(n+2)x=\cos x$

Solution:– $LHS = \sin(n+1)x\sin(n+2)x+\cos(n+1)x\cos(n+2)x$

$=\cos(n+1)x\cos(n+2)x+\sin(n+1)x\sin(n+2)x$

Using formula

$\cos (x-y)=\cos x\cos y+\sin x\sin y$

Hence,

$=\cos[(n+1)x-(n+2)x]$

$=\cos[nx+x-nx-2x]$

$=\cos(-x)$

$=\cos x=RHS$

Question 11: $\cos(\frac{3\pi}{4}+x)-\cos(\frac{3\pi}{4}-x)=-\sqrt{2}\sin x$

Solution: $LHS=\cos(\frac{3\pi}{4}+x)-\cos(\frac{3\pi}{4}-x)$

Using formula

$\cos (x-y)=\cos x\cos y+\sin x\sin y$

$\cos (x+y)=\cos x\cos y-\sin x\sin y$

NOW,

$=(\cos \frac{3\pi}{4}\cos x-\sin \frac{3\pi}{4}\sin x)-(\cos \frac{3\pi}{4}\cos x+\sin \frac{3\pi}{4}\sin x)$

$=\cos \frac{3\pi}{4}\cos x-\sin \frac{3\pi}{4}\sin x-\cos \frac{3\pi}{4}\cos x-\sin \frac{3\pi}{4}\sin x$

$=-2\sin \frac{3\pi}{4}\sin x$

$=-2(\frac{1}{\sqrt{2}})\sin x$

$=-\sqrt{2}\sin x=RHS$

Question 12: $\sin ^2 6 x-\sin ^2 4 x=\sin 2 x \sin 10 x$

Solution: $\text { L.H.S. }=\sin ^2 6 x-\sin ^2 4 x$

Using the formula

$\sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$\sin A-\sin B=2 \cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

So we get

$=(\sin 6 x+\sin 4 x)(\sin 6 x-\sin 4 x)$

$=\left[2 \sin \left(\frac{6 x+4 x}{2}\right) \cos \left(\frac{6 x-4 x}{2}\right)\right]\left[2 \cos \left(\frac{6 x+4 x}{2}\right) \cdot \sin \left(\frac{6 x-4 x}{2}\right)\right]$

$=(2 \sin 5 x \cos x)(2 \cos 5 x \sin x)$

$=(2 \sin 5 x \cos 5 x)(2 \sin x \cos x)$

$=\sin 10 \mathrm{x} \sin 2 \mathrm{x}=\text { RHS }$

Question 13: $\cos ^2 2 x-\cos ^2 6 x=\sin 4 x \sin 8 x$

Solution: $\text { L.H.S. }=\cos ^2 2 x-\cos ^2 6 x$

Using the formula

$\cos A+\cos B=2 \cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$\cos A-\cos B=-2 \sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

So we get

$=(\cos 2 x+\cos 6 x)(\cos 2 x-6 x)$

$=\left[2 \cos \left(\frac{2 x+6 x}{2}\right) \cos \left(\frac{2 x-6 x}{2}\right)\right]\left[-2 \sin \left(\frac{2 x+6 x}{2}\right) \sin \frac{(2 x-6 x)}{2}\right]$

$=[2 \cos 4 x \cos (-2 x)][-2 \sin 4 x \sin (-2 x)]$

$=[2 \cos 4 x \cos 2 x][-2 \sin 4 x(-\sin 2 x)]$

$=(2 \sin 4 x \cos 4 x)(2 \sin 2 x \cos 2 x)$

$=\sin 8 x \sin 4 x=\text { RHS }$

Question 14: $\sin 2 x+2 \sin 4 x+\sin 6 x=4 \cos ^2 x \sin 4 x$

Solution: $\text { L.H.S. }=\sin 2 x+2 \sin 4 x+\sin 6 x$

$=[\sin 2 x+\sin 6 x]+2 \sin 4 x$

Using the formula

$\sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$=\left[2 \sin \left(\frac{2 x+6 x}{2}\right) \cos \left(\frac{2 x-6 x}{2}\right)\right]+2 \sin 4 x$

$=2 \sin 4 x \cos (-2 x)+2 \sin 4 x$

$=2 \sin 4 x \cos 2 x+2 \sin 4 x$

Taking common terms

$=2 \sin 4 x(\cos 2 x+1)$

Using the formula

$=2 \sin 4 x\left(2 \cos ^2 x-1+1\right)$

$=2 \sin 4 x\left(2 \cos ^2 x\right)$

$=4 \cos ^2 x \sin 4$

$=\text { R.H.S. }$

Question 15: $\cot 4 x(\sin 5 x+\sin 3 x)=\cot x(\sin 5 x-\sin 3 x)$

Solution: $\text { LHS }=\cot 4 x(\sin 5 x+\sin 3 x)$

$=\frac{\cos 4 x}{\sin 4 x}\left[2 \sin \left(\frac{5 x+3 x}{2}\right) \cos \left(\frac{5 x-3 x}{2}\right)\right]$

Using the formula

$sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right) .$

$=\left(\frac{\cos 4 x}{\sin 4 x}\right)[2 \sin 4 x \cos x]$

$=2 \cos 4 x \cos \mathrm{x}$

Similarly

$\text { R.H.S. }=\cot x(\sin 5 x-\sin 3 x)$

$=\frac{\cos x}{\sin x}\left[2 \cos \left(\frac{5 x+3 x}{2}\right) \sin \left(\frac{5 x-3 x}{2}\right)\right]$

Using the formula

$\sin A-\sin B=2 \cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

$=\frac{\cos x}{\sin x}[2 \cos 4 x \sin x]$

$=2 \cos 4 \mathrm{x} \cos \mathrm{x}$

Hence, LHS $=$ RHS.

Question 16: $\frac{\cos 9 x-\cos 5 x}{\sin 17 x-\sin 3 x}=-\frac{\sin 2 x}{\cos 10 x}$

Solution: $\text { L.H.S }=\frac{\cos 9 x-\cos 5 x}{\sin 17 x-\sin 3 x}$

Using the formula

$\cos A-\cos B=-2 \sin \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

$\sin A-\sin B=2 \cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

$=\frac{-2 \sin \left(\frac{9 x+5 x}{2}\right) \cdot \sin \left(\frac{9 x-5 x}{2}\right)}{2 \cos \left(\frac{17 x+3 x}{2}\right) \cdot \sin \left(\frac{17 x-3 x}{2}\right)}$

$=\frac{-2 \sin 7 x \cdot \sin 2 x}{2 \cos 10 x \cdot \sin 7 x}$

$=-\frac{\sin 2 x}{\cos 10 x}$

$=\text { RHS }$

Question 17: $\frac{\sin 5 x+\sin 3 x}{\cos 5 x+\cos 3 x}=\tan 4 x$

Solution: $\text { L.H.S. }=\frac{\sin 5 x+\sin 3 x}{\cos 5 x+\cos 3 x}$

Using the formula

$\sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$\cos A+\cos B=2 \cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$=\frac{2 \sin \left(\frac{5 x+3 x}{2}\right) \cdot \cos \left(\frac{5 x-3 x}{2}\right)}{2 \cos \left(\frac{5 x+3 x}{2}\right) \cdot \cos \left(\frac{5 x-3 x}{2}\right)}$

$=\frac{2 \sin 4 x \cdot \cos x}{2 \cos 4 x \cdot \cos x}$

$=\frac{\sin 4 x}{\cos 4 x}$

$=\tan 4 x =\text { RHS }$

Question 18: $\frac{\sin x-\sin y}{\cos x+\cos y}=\tan \frac{x-y}{2}$

Solution: $\text { L.H.S. }=\frac{\sin x-\sin y}{\cos x+\cos y}$

Using the formula

$\sin A-\sin B=2 \cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A-B}{2}\right)$

$\cos A+\cos B=2 \cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$=\frac{2 \cos \left(\frac{x+y}{2}\right) \cdot \sin \left(\frac{x-y}{2}\right)}{2 \cos \left(\frac{x+y}{2}\right) \cdot \cos \left(\frac{x-y}{2}\right)}$

$=\frac{\sin \left(\frac{x-y}{2}\right)}{\cos \left(\frac{x-y}{2}\right)}$

$=\tan \left(\frac{x-y}{2}\right)=\text { RHS }$

Question 19: $\frac{\sin x+\sin 3 x}{\cos x+\cos 3 x}=\tan 2 x$

Solution: L.H.S. $=\frac{\sin x+\sin 3 x}{\cos x+\cos 3 x}$

Using the formula

$\sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$\cos A+\cos B=2 \cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$=\frac{2 \sin \left(\frac{x+3 x}{2}\right) \cos \left(\frac{x-3 x}{2}\right)}{2 \cos \left(\frac{x+3 x}{2}\right) \cos \left(\frac{x-3 x}{2}\right)}$

$=\frac{\sin 2 x}{\cos 2 x}$

$=\tan 2 \mathrm{x}= RHS$

Question 20: $\frac{\sin x-\sin 3 x}{\sin ^2 x-\cos ^2 x}=2 \sin x$

Solution: $\text { L.H.S. }=\frac{\sin x-\sin 3 x}{\sin ^2 x-\cos ^2 x}$

Using the formula

$\sin A-\sin \mathrm{B}=2 \cos \left(\frac{\mathrm{A}+\mathrm{B}}{2}\right) \sin \left(\frac{\mathrm{A}-\mathrm{B}}{2}\right)$

$\cos ^2 A-\sin ^2 A=\cos 2 A$

$=\frac{2 \cos \left(\frac{x+3 x}{2}\right) \sin \left(\frac{x-3 x}{2}\right)}{-\cos 2 x}$

$=\frac{2 \cos 2 x \sin (-x)}{-\cos 2 x}$

$=-2(-\sin x)$

$=2 \sin x = RHS$

Question 21: $\frac{\cos 4 x+\cos 3 x+\cos 2 x}{\sin 4 x+\sin 3 x+\sin 2 x}=\cot 3 x$

Solution: $\text { L.H.S. }=\frac{\cos 4 x+\cos 3 x+\cos 2 x}{\sin 4 x+\sin 3 x+\sin 2 x}$

$=\frac{(\cos 4 x+\cos 2 x)+\cos 3 x}{(\sin 4 x+\sin 2 x)+\sin 3 x}$

Using the formula

$\cos A+\cos B=2 \cos \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$\sin A+\sin B=2 \sin \left(\frac{A+B}{2}\right) \cos \left(\frac{A-B}{2}\right)$

$=\frac{2 \cos \left(\frac{4 x+2 x}{2}\right) \cos \left(\frac{4 x-2 x}{2}\right)+\cos 3 x}{2 \sin \left(\frac{4 x+2 x}{2}\right) \cos \left(\frac{4 x-2 x}{2}\right)+\sin 3 x}$

$=\frac{2 \cos 3 x \cos x+\cos 3 x}{2 \sin 3 x \cos x+\sin 3 x}$

$=\frac{\cos 3 x(2 \cos x+1)}{\sin 3 x(2 \cos x+1)}$

$=\cot 3 x = RHS$

Question 22: $\cot x \cot 2 x-\cot 2 x \cot 3 x-\cot 3 x \cot x=1$

Solution: $\text { LHS }=\cot x \cot 2 x-\cot 2 x \cot 3 x-\cot 3 x \cot x$

$=\cot x \cot 2 x-\cot 3 x(\cot 2 x+\cot x)$

$=\cot x \cot 2 x-\cot (2 x+x)(\cot 2 x+\cot x)$

Using the formula

$\cot (\mathrm{A}+\mathrm{B})=\frac{\cot \mathrm{A} \cot \mathrm{B}-1}{\cot \mathrm{A}+\cot \mathrm{B}}$

$=\cot x \cot 2 \mathrm{x}-\left[\frac{\cot 2 \mathrm{x} \cot \mathrm{x}-1}{\cot x+\cot 2 \mathrm{x}}\right](\cot 2 \mathrm{x}+\cot \mathrm{x})$

$=\cot x \cot 2 x-(\cot 2 x \cot x-1)$

$=1 = RHS$

Question 23: $\tan 4 x=\frac{4 \tan x\left(1-\tan ^2 x\right)}{1-6 \tan ^2 x+\tan ^4 x}$

Solution: $\text { LHS }=\tan 4 x=\tan 2(2 x)$

By using the formula

$\tan 2 A=\frac{2 \tan A}{1-\tan ^2 A}$

$=\frac{2 \tan 2 x}{1-\tan ^2(2 x)}$

$=\frac{2\left(\frac{2 \tan x}{1-\tan ^2 x}\right)}{1-\left(\frac{2 \tan x}{1-\tan ^2 x}\right)^2}$

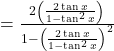
$=\frac{\left(\frac{4 \tan x}{1-\tan ^2 x}\right)}{\left[1-\frac{4 \tan ^2 x}{\left(1-\tan ^2 x\right)^2}\right]}$

Taking LCM

$=\frac{\left(\frac{4 \tan x}{1-\tan ^2 x}\right)}{\left[\frac{\left(1-\tan ^2 x\right)^2-4 \tan ^2 x}{\left(1-\tan ^2 x\right)^2}\right]}$

$=\frac{4 \tan x\left(1-\tan ^2 x\right)}{\left(1-\tan ^2 x\right)^2-4 \tan ^2 x}$

$=\frac{4 \tan x\left(1-\tan ^2 x\right)}{1+\tan ^4 x-2 \tan ^2 x-4 \tan ^2 x}$

$=\frac{4 \tan x\left(1-\tan ^2 x\right)}{1-6 \tan ^2 x+\tan ^4 x} =RHS$

Question 24: $\cos 4 x=1-8 \sin ^2 x \cos ^2 x$

Solution: $\text { LHS }=\cos 4 \mathrm{x}$

$=\cos 2(2 x)$

Using the formula

$\cos 2 A=1-2 \sin ^2 A$

$=1-2 \sin ^2 2 x$

Again by using the formula

$\sin 2 A=2 \sin A \cos A$

$=1-2(2 \sin x \cos x)^2$

$=1-8 \sin ^2 x \cos ^2 x = R.H.S.$

Question 25: $\cos 6 x=32 \cos ^6 x-48 \cos ^4 x+18 \cos ^2 x-1$

Solution: L.H.S. $=\cos 6 x$

$=\cos 3(2 x)$

Using the formula

$\cos 3 A=4 \cos ^3 A-3 \cos A$

$=4 \cos ^3 2 x-3 \cos 2 x$

Again by using formula

$\cos 2 x=2 \cos ^2 x-1$

$=4\left[\left(2 \cos ^2 x-1\right)^3-3\left(2 \cos ^2 x-1\right)\right.$

$=4\left[\left(2 \cos ^2 x\right)^3-(1)^3-3\left(2 \cos ^2 x\right)^2+3\left(2 \cos ^2 x\right)\right]-6 \cos ^2 x+3$

$=4\left[8 \cos ^6 x-1-12 \cos ^4 x+6 \cos ^2 x\right]-6 \cos ^2 x+3$

By multiplication

$=32 \cos ^6 x-4-48 \cos ^4 x+24 \cos ^2 x-6 \cos ^2 x+3$

$=32 \cos ^6 x-48 \cos ^4 x+18 \cos ^2 x-1=R.H.S.$

https://gmath.in/ex-3-2-trigonomety-ncert-maths-solution-class-11/

EXERCISE 3.3(Trigonometric Function)

Leave a Comment Cancel reply